Introduction
For the academic year 2022–2023, the Central Board of Secondary Education (CBSE) has released the Class 10 rationalized syllabus. Around 20-30%, class 10th math’s syllabus has been reduced. With the emergence of new technological requirements, the math’s pattern has undergone some changes. The secondary curriculum aligned main goal is to improve students’ ability to use mathematics to solve problems in daily life. The proposed curriculum covers a variety of topics, such as the study of coordinate geometry, graphs, statistics, algebra, geometry, and trigonometry.
The CBSE Class 10th Maths syllabus lays the foundation for important board and competitive exams in the coming years. If you want a step-by-step guide to prepare for class 10th math’s exam, then read this.
A syllabus is a type of instructional tool that establishes expectations for what will occur throughout the entire course session. It specifies the subjects and ideas that will form the basis of the final exam questions for the students. The syllabus outlines the functions and concepts used to evaluate students’ performance, serving as a contract between students and teachers.
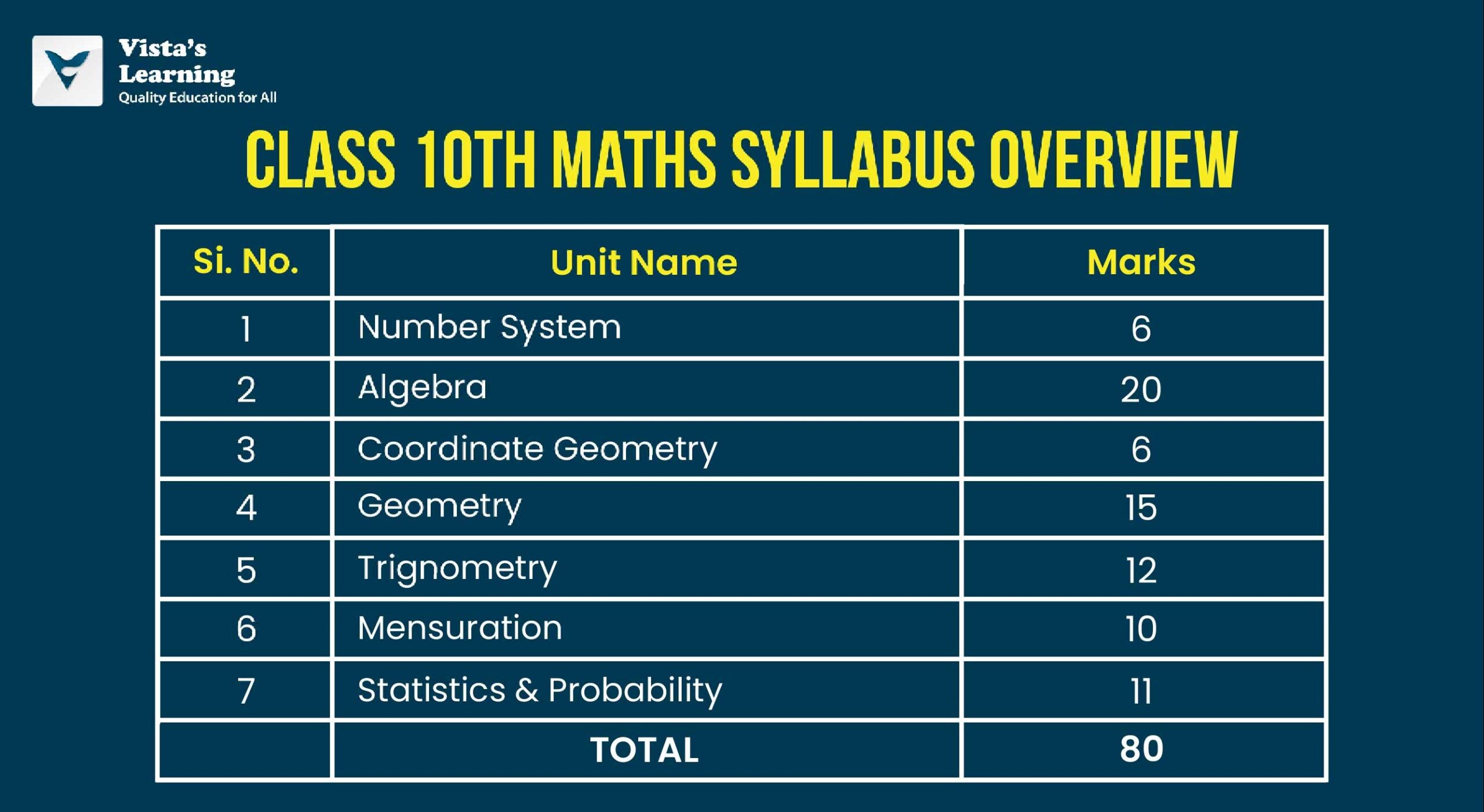
Class 10th Math’s Syllabus Overview
First, let’s examine the course structure to understand the importance of each component and topic this year.

15 Chapters of Class 10th Math’s Syllabus 2023-2024:
Unit 1: Number System
The number system, or numeral system, identifies and expresses numbers. It uniquely represents each number while defining their geometric and algebraic structure. It gives us the ability to carry out various mathematical operations, including addition, subtraction, and division.
Rational number:
Rational numbers are those that can be expressed as a fraction, a positive number, a negative number, or a zero. If y is not zero, it can be written as x/y.
Example: For instance, 5/3 is a rational number. It means that an integer 5 has been divided by an extra added integer 3.
Irrational number:
An irrational number is any number that is not rational. It is a non-repeating, non-terminating decimal that cannot represent a ratio of two integers.
Unit 2: Algebra
- Overview of algebra
- Expansion and factorization of algebraic expressions.
- Quadratic equations and their solutions.
A significant area of mathematics called algebra finds critical use in the study of engineering and technology. It deals with the rules for manipulating symbols known as variables, which represent the value of any quantity at any time.
Expansion and factorisation of algebraic expressions:
Factorization refers to finding the factors of an algebraic expression by identifying two or more expressions whose product equals the given expression. It simply means representing a number as the product of two other numbers. Similarly, factors form the product of algebraic expressions. The only distinction is that an algebraic expression here combines addition or subtraction with arithmetic operations like numbers and variables.
Quadratic equations and their solutions:
In mathematics, a quadratic equation is a second-degree polynomial with the standard form ax2 + bx + c = 0, where a, b, and c are numerical coefficients and a is not equal to 0.
Unit 3: Coordinate Geometry
A plane is a flat surface that extends infinitely in all directions. With the development of coordinate geometry, locating a point on a plane has become easier. Any point on the plane can be located using the two numbers of coordinate geometry. Tell us more, please!
A branch of geometry known as coordinate geometry uses an ordered pair of numbers, or coordinates, to define the location of points on a plane.
Understanding the concept of graphs:
Coordinate graphs use two perpendicular number lines called coordinate axes. The horizontal axis is the x-axis, while the vertical axis is the y-axis. The origin, where both axes meet, is identified by the coordinates (0,0).
An x‐y coordinate graph.
Unit 4: Geometry
The area of mathematics known as geometry is concerned with the dimensions, sizes, shapes, and angles of a wide range of everyday objects.The term Geometry comes from two Ancient Greek words meaning “measurement” and “Earth.
Flat shapes include 2-dimensional shapes like triangles, squares, rectangles, and circles. Three-dimensional shapes are cubes, cuboids, cones, etc referred to as solids in solid geometry. The coordinate geometry of points, lines, and planes forms the foundation of fundamental geometry.
Triangle & their properties:
In mathematics, triangles are considered to be a part of geometry. In other words, it has a specific shape that sets it apart from other geometrical shapes you encounter quite often. A triangle, however, is primarily a closed polygon with three straight sides.
Types of Triangle:
Scalene Triangle
Isosceles Triangle
Equilateral Triangle
Acute Angle Triangle
Right Angle Triangle
Obtuse Angle Triangle
Properties of triangle:
- The sum of a triangle’s exterior angles is always 360 degrees.
- Consecutive interior and exterior angles added together are supplemental.
- The shortest side always opposes the interior angle with the smallest measure. The largest interior angle is always opposite the longest side, in a similar manner.
Circles & their properties:
A circle can be made by moving along with a moving point in a plane while keeping a fixed distance from another point. The Greek word kirkos, which means hoop or ring, is where the word circle gets its name.
Properties of Circles Related to Lines
Chord
Tangent
Properties of Circles Related to angles
Inscribed Angle
Central Angle
Important Formulas:
Perimeter–Circle Formula
Area – Circle Formula
Unit 5: Trigonometry
The most crucial mathematical concept is trigonometry. It deals with the right-angled triangle’s sides and angles. It is extremely important in almost every field, including physics, criminology, satellite navigation, medical imaging, and many others. Finding the angles or sides of a right-angled triangle is done using trigonometry.
Trigonometric Ratios:
For the right ΔPQR, right-angled at ∠Q, the trigonometric ratios of the ∠P are as follows:
- sin P=opposite side/hypotenuse= QR/PR
- cos P=adjacent side/hypotenuse= PQ/PR
- tan P=opposite side/adjacent side= QR/PQ
- Cosec P=hypotenuse/opposite side= PR/QR
- sec P=hypotenuse/adjacent side= PR/PQ
- cot P=adjacent side/opposite side= PQ/QR
Trigonometric identities:
The equations that include trigonometric functions like sine, cosine, tangent, etc. There are essentially three trigonometric identities taught in class 10 and covered in the trigonometry chapter. They are:
- Cos2 θ + Sin2 θ = 1
- 1 + Tan2 θ = Sec2 θ
- 1 + Cot2 θ = Cosec2 θ
Unit 6: Mensuration
Mensuration studies the measurement of geometric objects and their attributes, such as length, volume, shape, and surface area.The mathematical techniques used here are the mensuration formulas. Measurements are used to provide information about an object’s height, width, depth, perimeter, area, and volume. Mensuration describes the characteristics and formulas of a variety of geometrical shapes, including two-dimensional shapes.
Formula for Volume of different shapes:
Sphere = (4/3) π x (radius)3
Prism or Cylinder = Base area x height
Pyramid or Cone (V) = (frac {1}{3}) x base area x height.
Unit 7: Statistics & Probability
Probability:
It is the possibility of any random event’s outcome. Identifying the likelihood that a specific event will occur is meant by this expression. When we toss a coin in the air, what are the chances of getting a head, for example? The answer is based on the number of possible outcomes. Either head or tail could come out of this situation. Therefore, the likelihood that the outcome will be head is 50%.
P(E) = n(E)/n(S)
Here,
n(S) = Total number of outcomes
Statistics:
The study of data gathering, analysis, and organization is known as statistics. A technique for gathering and analyzing data. This has numerous uses on both a small and large scale. Stats are used in all such data analysis, whether it be for studying a nation’s population or its economy.
Mean, mode & median:
The average of any data set is called the mean.
A data set’s mode is its most common number.
The middle value in a set of numbers is called the median.
Formulas of Mean and Median:
Mean = Sum of Observation/Total Number of Observations
If ‘n’ is odd: Median = (n+1/2)th term
If ‘n’ is even: Median = [(n/2)th term + (n/2 +1)th term]/2
Conclusion:
Studying class 10th math’s syllabus is never hard, if you focus on the core basics and attend every lecture sincerely. Class 10th boards is your new milestone and to achieve this, you need to study consistently with patience.
We Vista’s Learning
We at Vista’s Learning think that every student should be able to receive an affordable, high-quality education. For just Rs. 999, we have started offering online classes for students in grades 1 through 10 in order to accomplish this goal. Because of our entertaining co-curricular activity sessions, animated video lessons, and streamlined study materials, learning from Vista’s Learning is far too simple.
FAQs of Class 10th Math’s Syllabus
How many chapters are there in the 10th class math’s syllabus?
There are 15 chapters in CBSE class 10th math’s syllabus. These 15 chapters are grouped into 7 units: Number System, Algebra, Coordinate Geometry, Geometry, Trigonometry, Mensuration, and Statistics & Probability.
Is 10th Math’s Difficult?
CBSE Class 10th Math’s Syllabus is moderate but the CBSE class 10th paper is bit lengthy and it consumes more time to solve.
Which unit of class 10th math’s syllabus has the highest weightage?
Algebra has the highest weightage of 20 marks out of 80 marks. There are 4 chapters in Algebra that are: Polynomials, Pair of Linear Equations in two Variables, Quadratic Equations, and Arithmetic Progressions.
Which unit of class 10th math’s syllabus has the lowest weightage?
Number Systems has the lowest weightage of 6 marks out of 80 marks. There is only 1 chapter in Number Systems that is: Real Numbers.
Deleted part of the blog:
Question
In a seminar, there are 60, 84, and 108 participants in Hindi, English, and Mathematics, respectively. Determine the minimum number of rooms needed to seat an equal number of participants studying the same topic.
Solution
By prime factorisation
60=2*2*3*5
84=2*2*3*7
108=2*2*3*3*3
HCF =12
Rooms required= 60/12 + 84/12+108/12=5+7+9=21
